(solved)Question 4 SSC-CGL 2020 March 3 Shift 2

Question 4
SSC-CGL 2020 Mar 3 Shift 2

In-radius of a triangle is 3 cm. What is the perimeter if its area is 15 sq. cm?

Solution in Brief

$\displaystyle \therefore P = \frac{2\times \text{Area}}{\text{in-radius}}$

which $\displaystyle = \frac{2 \times 15}{3} = 10 \text{ cm}\:\underline{Ans}$

Additional Notes

Let us derive the above relation from fundamentals.

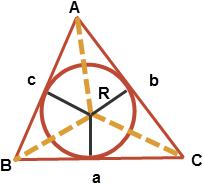
The triangle can be split into three triangles of areas:

$\displaystyle \frac12 a R$, $\displaystyle \frac12 b R$ and $\displaystyle \frac12 c R$

Adding these areas, the area of the triangle becomes

$\displaystyle A = \frac12 R(a + b + c)$.

Or, $\displaystyle 2A = R \times \text{perimeter!}$