(solved)Question 8 SSC-CGL 2020 March 4 Shift 2

Question 8
SSC-CGL 2020 Mar 4 Shift 2

PRT is a tangent to a circle with center O touching the circle at R. Diameter SQ is produced to meet P. If angle PRQ = 28 degree, then find angle SPR.

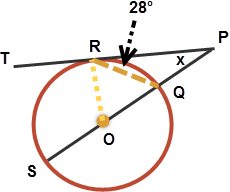
Solution in Detail

by exterior angle of $\displaystyle \Delta {PQR}$,

$\displaystyle \angle {OQR} = x + 28$

Observe $\displaystyle \Delta {OQR}$ is isosceles:

$\displaystyle \therefore \angle{ORQ} = \angle {OQR} = x + 28$

But radius $\displaystyle OR \perp \text{tangent PRT} $

$\displaystyle \therefore \angle{ORQ} + \angle{QRP} = 90$

$\displaystyle \therefore (x + 28) + 28 = 90$

$\displaystyle \implies x = 34\degree \:\underline{Ans}$