(solved)Question 10 SSC-CGL 2020 March 3 Shift 1

Question 10
SSC-CGL 2020 Mar 3 Shift 1

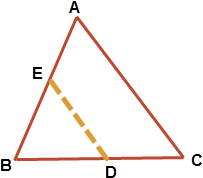
ABC is a triangle with an area of 44 sq. cm. If D and E are the midpoints of BC and AB respectively, then the area of triangle BDE is?

Solution in Short

Triangles BDE and ABC are similar. The ratio of their sides is 1 : 2, so the ratio of their areas is squared to 1 : 4, which gives the area of BDE as 44/4 = 11 sq. cm answer!

Solution in Detail

Let us observe the triangles ABC and BDE

[1] D is midpoint of BC, so $\displaystyle \frac{\text{BD}}{\text{BC}}= \frac{1}{2}$

[2] E is midpoint of AB, so $\displaystyle \frac{\text{BE}}{\text{AB}}= \frac{1}{2}$

[3] Angle B is common

$\displaystyle \therefore \text{by SAS, } \Delta ABC \sim \Delta BDE$

$\displaystyle \implies \frac{\text{ar(BDE)}}{\text{ar(ABC)}} = \bigg(\frac{1}{2}\bigg)^2$

$\displaystyle \implies \text{ar(BDE)} = \frac{1}{4} \times 44 $

$\displaystyle \therefore \text{ar(BDE)} = 11 \text{ sq. cm Ans!}$