(solved)Question 10 SSC-CGL 2018 June 4 Shift 2

Question 10
SSC-CGL 2018 June 4 Shift 2

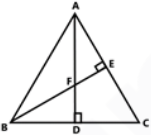
In ΔABC, AD ⊥ BC and BE ⊥ AC. AD and BE cut each other at F. If BF = AC, what will be the measure of ∠ABD?

1. 60°
2. 45°
3. 50°
4. 70°

Solution in Short

First use the facts that AD ⊥ BC and BE ⊥ AC to prove that the triangles BFD and FAE are right angled and also have their other two respective angles equal.

Then use the above conculsion to prove that the triangles BFD and ADC are congruent because BF = AC is given. Because of this congruency, BD and AD are equal, which proves that the triangle ABD is a right isosceles triangle, with angle ABD of 45 degrees.

Solution in Detail

[1] $\displaystyle \Delta DBF$ and $\displaystyle \Delta FAE$ are right angled.

[2] their angles at F are equal, being vertically opposite.

[So, ]their third angles are equal -

$\displaystyle \therefore \angle {DBF} = \angle {FAE} \text{ . . . (1)}$

Next take $\displaystyle \Delta BDF$ and $\displaystyle \Delta ADC$

[1]Both are right angled.

[2]By $\displaystyle \text{(1)}$ above, $\displaystyle \angle {DBF} = \angle {FAE}$

[3]Given that the sides $\displaystyle \overline{\text{BF}} = \overline{\text{AC}}$

Hence, by ASA rule $\displaystyle \Delta BDF \cong \Delta ADC$

$\displaystyle \implies \overline{\text{BD}} = \overline {\text{AD}}$

$\displaystyle \implies \Delta ABD$ is isosceles

But $\displaystyle \Delta ABD$ is given right angled.

Hence the equal angles are $\displaystyle 45\degree$ each.

$\displaystyle \implies \angle{\text{ABD}} = 45\degree \:\underline{Ans}$

This Blog Post/Article "(solved)Question 10 SSC-CGL 2018 June 4 Shift 2" by Parveen is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License.