(solved)Question 10 SSC-CGL 2020 March 4 Shift 1

Question 10
SSC-CGL 2020 Mar 4 Shift 1

In triangle ABC, ∠C = 68°, ∠B = 32°. The external angle bisectors of ∠B and ∠C meet at point O. Find ∠BOC.

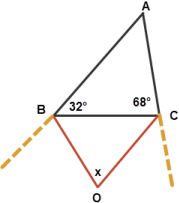
Solution inBrief

∠A = 180 - (32 + 68) = 80. By a theorem in geometry, ∠BOC = 90 - (∠A/2). So ∠BOC = 90 - 80/2 = 50 degree answer!

Solution in Detail

BO is angle bisector, therefore,

$\displaystyle \angle {OBC} = \frac{180 - 32}{2} = 74\degree$

Similarly, we can deduce that

$\displaystyle \angle {BCO} = \frac{180 - 68}{2} = 56\degree$

From $\displaystyle \Delta OBC$ we get

$\displaystyle x + 74 + 56 = 180 $

$\displaystyle \therefore x = 50 \degree\:\underline{Ans}$